neufer wrote:NoelC wrote:Markus Schwarz wrote:
Same math but I used the Wikipedia size: 8 × 6 arcmins and assumed that "nose" to "mane" was the 8 arcmins.
An astronomer friend of mine once let on that pretty much all measurements in the cosmos are quite inexact - essentially best guesses.
That said, I just retraced my steps. Here's what I did... Note the declination measurements for the two points I've chosen in these sky chart program screen grabs:
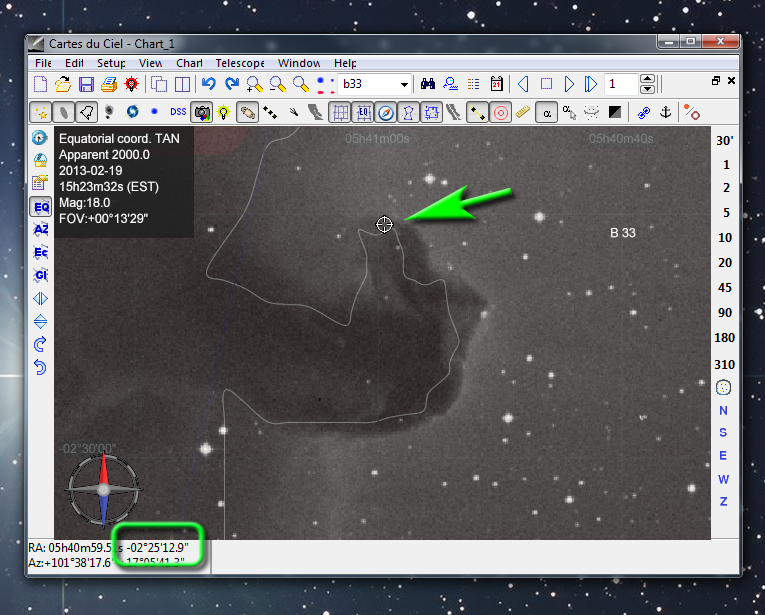
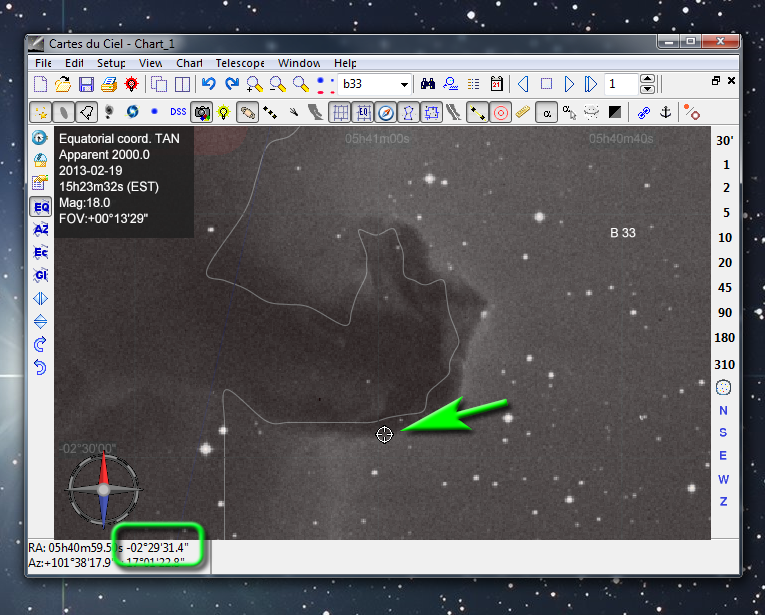
This time I retrieved the Digitized Sky Survey image, which the chart program overlaid for me. Prior to this I had measured to the chart outline, which as you can see is a bit smaller than the extents of the actual nebula.
Subtracting the two declination measurements above, we get 4' 18.5" difference, or roughly 4.31'.
Since the horsehead is not far from the celestial equator (about 2.5 degrees), I originally roughly equated the declination difference to the angular size of the object. In fact, the angular size of an object will be progressively larger than the declination difference for objects as we move away from the equator and closer to the pole, so let's push the actual size a bit up further to 4.31 * (90 / 87.5), or 4.43 minutes of arc.
1500 * tan(4.43 / 60) == 1.9 light-years. This is assuming the 1500 light-year distance is correct and expressing the number in 2 significant digits makes sense, but in fact one can find estimates that go lower and higher (I found more lower than higher).
So let's say a "best" guess is 2ish light years, nose to mane, or about 1300ish times the diameter of the orbit of pluto. Looked at another way, if the horsehead were displayed in an image where it was 1300 pixels wide, mostly filling a monitor screen, the space our solar system occupies (to the orbit of Pluto, to which our fastest spacecraft take
years to reach) would be 1 pixel.
That's one big horse!

-Noel